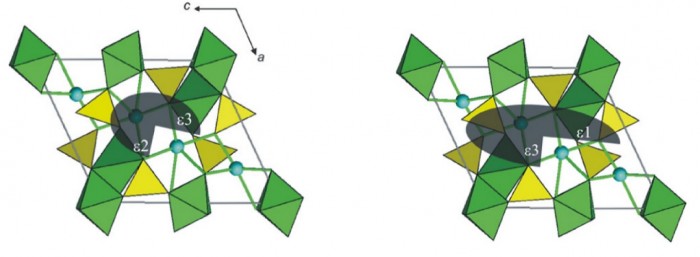
The thermo-elastic behavior of a natural epidote [Ca1.925 Fe0.745Al2.265Ti0.004Si3.037O12(OH)] has been investigated up to 1,200 K (at 0.0001 GPa) and 10 GPa (at 298 K) by means of in situ synchrotron powder diffraction. No phase transition has been observed within the temperature and pressure range investigated. P–V data fitted with a third-order Birch–Murnaghan equation of state (BM-EoS) give V 0 = 458.8(1)Å3, K T0 = 111(3) GPa, and K′ = 7.6(7). The confidence ellipse from the variance–covariance matrix of K T0 and K′ from the least-square procedure is strongly elongated with negative slope. The evolution of the “Eulerian finite strain” vs “normalized stress” yields Fe(0) = 114(1) GPa as intercept values, and the slope of the regression line gives K′ = 7.0(4). The evolution of the lattice parameters with pressure is slightly anisotropic. The elastic parameters calculated with a linearized BM-EoS are: a 0 = 8.8877(7) Å, K T0(a) = 117(2) GPa, and K′(a) = 3.7(4) for the a-axis; b 0 = 5.6271(7) Å, K T0(b) = 126(3) GPa, and K′(b) = 12(1) for the b-axis; and c 0 = 10.1527(7) Å, K T0(c) = 90(1) GPa, and K’(c) = 8.1(4) for the c-axis [K T0(a):K T0(b):K T0(c) = 1.30:1.40:1]. The β angle decreases with pressure, βP(°) = βP0 −0.0286(9)P +0.00134(9)P 2 (P in GPa). The evolution of axial and volume thermal expansion coefficient, α, with T was described by the polynomial function: α(T) = α0 + α1 T −1/2. The refined parameters for epidote are: α0 = 5.1(2) × 10−5 K−1 and α1 = −5.1(6) × 10−4 K1/2 for the unit-cell volume, α0(a) = 1.21(7) × 10−5 K−1 and α1(a) = −1.2(2) × 10−4 K1/2 for the a-axis, α0(b) = 1.88(7) × 10−5 K−1 and α1(b) = −1.7(2) × 10−4 K1/2 for the b-axis, and α0(c) = 2.14(9) × 10−5 K−1 and α1(c) = −2.0(2) × 10−4 K1/2 for the c-axis. The thermo-elastic anisotropy can be described, at a first approximation, by α0(a): α0(b): α0(c) = 1 : 1.55 : 1.77. The β angle increases continuously with T, with βT(°) = βT0 + 2.5(1) × 10−4 T + 1.3(7) × 10−8 T 2. A comparison between the thermo-elastic parameters of epidote and clinozoisite is carried out.